(Photo by Cliff Welch/Icon Sportswire)
Today I want to talk about my latest preoccupation– the concept of Effective Velocity. While I’ll freely admit I’m still a novice when it comes to the idea as a whole (and there is a WHOLE lot), the basics have begun to resonate in my head.
Effective Velocity is a tool a pitcher can use against a hitter to gain an advantage, pioneered and championed by Mr. Perry Husband. Here is a great explanation Jason Turbow came up with in an article he wrote several years ago:
It hinges on response time. Husband’s model is based on the arc of hitters’ swings, and the understanding that bats must move farther to reach pitches on the inner part of the plate than on the outside edge. Put another way, a batter can hit an outside fastball as it crosses the plate, but to make solid contact with an inside fastball, he must reach it much sooner — up to 2 feet in front of the plate — which requires the hitter to move the bat a greater distance in less time. With this detail in mind, it makes sense to build an approach based not on a pitch’s radar speed, but how quickly the man standing in the batter’s box can react to it.
For reference, here is an EV chart that can be applied to a right-handed hitter (from the pitcher’s view); simply reverse the chart for a lefty.
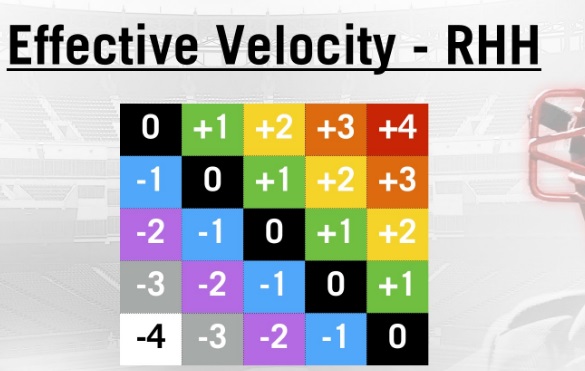
Chart 1
I’m going to focus on this three-pitch sequence featuring Tampa Bay Rays starter Tyler Glasnow and his duel with Cleveland Indians hitter Edwin Encarnacion. Besides the fact that its a great example, I am also fortunate enough to make use of one of the best Major League Baseball camera angles according to site owner Nick Pollock (Spoiler: He’s not wrong!).
During their September 11th matchup in Tampa, Glasnow needed just three pitches to strike Encarnacion out. While on the surface it would appear that this is nothing more than a fairly accustomed event, I want to divulge that there is much more going on.
First, let’s look at the pitch sequence. Below is pitch number one– a 96 MPH four-seam fastball.
https://gfycat.com/terribleminorkoi
Glasnow puts the pitch up and in to Encarnacion, which he fouls off. While the radar gun reads 96 MPH, the Effective Velocity of the pitch is actually closer to 99/100 EvMPH. From this point on, anything along this tunnel (or trajectory) will be in line with the aforementioned EV. Meaning, A few miles per hour off on the next pitch could cause Encarnacion to be early. Or, a pitch placed in a location farther away will ‘appear’ even slower to the hitter and throw him off.
Why is this? Well, it’s because of the impact on timing and reach of the hitter. A ball moving inside to a hitter requires him to react quicker because of the body and bat/swing adjustments needed to make solid (barrelled) contact; he would have to time and react to the pitch the same way he would with a 100 MPH fastball, even though its actually only 96 MPH. Let’s keep in mind EV isn’t solely dependent on swing-and-misses necessarily, but to induce bad or weak contact as well
Here is pitch number two– a 97 MPH fastball that is located middle-up inside the strike zone. Now it’s up to Encarnacion to add (or subtract) the EV to allow him to be perfectly on time with his swing.
https://gfycat.com/pleasingpoorbufflehead-cleveland-indians-baseball
So we have a pitch that is +1 MPH faster than the previous one read by the radar gun. But, according to Effective Velocity (see chart 1), it isn’t 97 MPH; its actually closer to 98 EvMPH, which is a -2MPH spread as opposed to a +1 MPH spread in favor of pitch two. Once again, Encarnacion fouls the ball off. This time (and its hard to see), he’s a little behind and under the pitch as opposed to the previous pitch where Encarnacion was simply under and perhaps ever so slightly late. At this point, you would think that Encarnacion has the pitched timed; his swing path and body language might agree.
It must also be noted that, according to Mr. Husband, 50% of hard contact comes from pitches thrown within 6 EvMPH of each other. Here we have the other 50% following pitch two. If a pitcher wants to be successful when applying EV, they need to take into consideration their ‘actual’ velocity and apply it to the planned location of the next pitch.
Pitch number three is an awesome 85 MPH slider down and away; the ‘freeze pitch’. It’s my understanding that it’s called a ‘freeze pitch’ because the hitter expects a certain pitch (and velocity) but is thrown off by the break/movement that varies from the last pitch or two; they check-swing or hold off entirely.
https://gfycat.com/requiredlikelygoa-cleveland-indians-baseball
Now we can see how Effective Velocity takes hold of the batter. Encarnacion, under the EV spell of a 99-100 EvMPH fastball, sees the slider coming at him at the same trajectory as the previous pitch. But this time it comes much slower and utilizes its spin rate (3214 RPM!) to hold the pitch in the tunnel and break right around the commit point; Glasnow couldn’t have thrown that slider any better. As we saw, Encarnacion offered, then tried to hold up because he was fooled by the pitch tunnel and movement of the slider. He’s called out on strikes.
Let’s see that tunnel in action.
https://gfycat.com/bestelaboratearachnid
From the freeze-frame below, we see both pitches following the same trajectory. However, the slider (yellow trail) had an extension point nearly a full inch longer than the previous fastball. That doesn’t matter too much so long as the path to home plate is the same (as the previous pitch).

And there you have it; Effective Velocity in its simplest and most effective form. Changing speeds and locations/eye level is nothing new; now we have a principle (EV) in place to quantify it. I can’t speak for Glasnow or even imply he is aware of EV, but what he’s done during this at-bat encompasses the concept.
The next time you find yourself (or someone else) trying to wrap your head around why a hitter did or did not swing at a certain pitch, the answer is likely as simple as “…because of Effective Velocity”.

Well done………….. The slider is an Ev ‘Pressure Pitch’, rather than a ‘Freeze Pitch’ which would be if the slider was outside the Ev Tunnel and then moving back to the strike zone. The Pressure slider shares the Ev Tunnel with the fastball more than 1/3 (probably more like halfway or .200 seconds) of the flight and then begins moving away, after the hitter has had to commit to the swing. The fastball is gaining EvMPH and the slider is losing EvMPH, maximizing the effect of both pitches on reaction time. Pitchers are beginning to understand and things will get much more difficult.
Stealing has become far more difficult because pitchers began measuring their time to the plate, catchers improving catch and release time and coaches focusing on it at the amateur level. In a similar way, Ev is the metric for maximizing deception and minimizing hard contact.
Always appreciate the feedback, Perry!
Thаnk уou for shɑring your info. I really aρpreϲiate your effortѕ
and I am waiting foг your further poѕt thank you once again.